A binary black hole system is constructed on a Cartesian grid from data stored in a file resulting from a computation by Grandclement, Gourgoulhon and Bonazzola, Phys. Rev. D 65, 044021 (2002).
All the quantities are in units derived from the length
scale defined by the coordinate radius 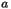 of black hole 1 apparent horizon
(throat).
of black hole 1 apparent horizon
(throat).
Importation of Lorene data is performed by means of the constructor
Bin_BH::Bin_BH(int, const double*, const double*, const double*, const char*).
This constructor takes general arrays for the location of the Cartesian coordinates
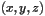 , i.e. it does not assume that the grid is a uniform one. Note also
that these arrays are 1-D, as well as all the metric fields,
in order to be use with any ordering of the 3-D storage.
, i.e. it does not assume that the grid is a uniform one. Note also
that these arrays are 1-D, as well as all the metric fields,
in order to be use with any ordering of the 3-D storage.
This class is very simple, with all data members being public. A typical example of use is the following one
* // Define the Cartesian grid by means of the arrays xg, yg, zg:
* for (int i=0; i<nb_points; i++) {
* xg[i] = ...
* yg[i] = ...
* zg[i] = ...
* }
*
* // Read the file containing the spectral data and evaluate
* // all the fields on the Cartesian grid :
*
* Bin_BH binary_system(nb_points, xg, yg, zg, fill, datafile) ;
*
* // Extract what you need :
*
* double* gamma_xx = binary_system.g_xx ; // metric coefficient g_xx
*
* double* shift_x = binary_system.beta_x ; // x comp. of shift vector
*
* ...
*
* // Save everything in an ASCII file :
*
* ofstream file_ini("ini.d") ;
* binary_system.save_form(file_ini) ;
* file_ini.close() ;
*
*
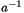 ]
]
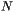 at the np grid points (1-D array)
at the np grid points (1-D array)
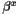 of the shift vector of corotating coordinates [unit:
of the shift vector of corotating coordinates [unit: 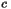 ]
]
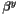 of the shift vector of corotating coordinates [unit:
of the shift vector of corotating coordinates [unit: 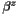 of the shift vector of corotating coordinates [unit:
of the shift vector of corotating coordinates [unit: 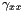 at the grid points (1-D array)
at the grid points (1-D array)
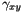 at the grid points (1-D array)
at the grid points (1-D array)
 at the grid points (1-D array)
at the grid points (1-D array)
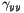 at the grid points (1-D array)
at the grid points (1-D array)
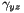 at the grid points (1-D array)
at the grid points (1-D array)
 at the grid points (1-D array)
at the grid points (1-D array)
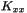 of the extrinsic curvature at the grid points (1-D array) [unit:
of the extrinsic curvature at the grid points (1-D array) [unit: 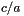 ]
]
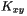 of the extrinsic curvature at the grid points (1-D array) [unit:
of the extrinsic curvature at the grid points (1-D array) [unit: 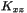 of the extrinsic curvature at the grid points (1-D array) [unit:
of the extrinsic curvature at the grid points (1-D array) [unit: 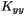 of the extrinsic curvature at the grid points (1-D array) [unit:
of the extrinsic curvature at the grid points (1-D array) [unit: 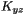 of the extrinsic curvature at the grid points (1-D array) [unit:
of the extrinsic curvature at the grid points (1-D array) [unit: 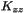 of the extrinsic curvature at the grid points (1-D array) [unit:
of the extrinsic curvature at the grid points (1-D array) [unit:  of the conformal factor
of the conformal factor 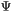 [unit:
[unit: 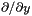 of the conformal factor
of the conformal factor 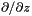 of the conformal factor
of the conformal factor 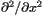 of the conformal factor
of the conformal factor  ]
]
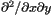 of the conformal factor
of the conformal factor  of the conformal factor
of the conformal factor 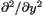 of the conformal factor
of the conformal factor 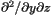 of the conformal factor
of the conformal factor  of the conformal factor
of the conformal factor